​Abstract: ​

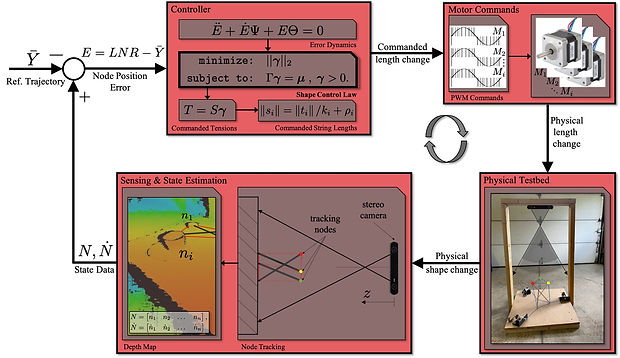
As we further extend our reach into outer space, there exists an unmet need for autonomous agents to carry out highly dexterous manipulation tasks such as on-orbit servicing and habitat construction. To be packaged efficiently for transport and autonomously deployed at a remote destination, these robotic mechanisms must be lightweight, yet highly articulated. Tensegrity structures, which comprise a continuous tendon network, are a suitable candidate for carrying out dexterous manipulation tasks in outer space. This thesis presentation focuses on controlling the shape of tensegrity structures by changing the tension in the supporting tendons.

        A vector-based approach is used to model the multi-body dynamics of tensegrity structures in a non-minimal coordinate system. This methodology is further extended to handle Class-k structures by modeling bar contact forces as Lagrange constraint forces. Leveraging the vector-based dynamics model, a state feedback controller is developed to regulate the shape of a tensegrity structure to a desired reference trajectory.

        The developed control law is implemented in simulation on several Class-1 and Class-k tensegrity structures. Then, a novel robotic manipulator is developed by using self-similar iterations to yield a structure that is both highly dexterous and lightweight, proving that the modeling and control framework can be used to design complex engineering structures. Finally, an experimental cylindrical triplex tensegrity structure is constructed and actuated based on the methods described. Through an open-loop demonstration, it is shown that the experimental structure serves as a suitable basis for testing future tensegrity control architectures.